Der Gesamtwirkungsquerschnitt einer Gammastrahlung mit einem Atom ist gleich der Summe aller drei genannten Teilquerschnitte : σ = σ f + σ C + σ p
- σ f – Photoelektrischer Effekt
- σ C – Compton-Effekt
- σ p – Paarbildung
Je nach Gammastrahlungsenergie und Absorbermaterial kann einer der drei Teilquerschnitte deutlich größer werden als die anderen beiden. Bei kleinen Werten der Gammastrahlenenergie dominiert der photoelektrische Effekt . Compton-Effekt dominiert bei mittleren Energien. Auch die Comptonstreuung nimmt mit abnehmender Ordnungszahl der Materie zu, daher ist das Dominanzintervall für leichte Kerne breiter. Schließlich dominiert bei hohen Energien die Erzeugung von Elektron-Positron-Paaren. Basierend auf der Definition des Wechselwirkungsquerschnitts kann die Abhängigkeit der Gammastrahlenintensität von der Dicke des Absorbermaterials abgeleitet werden. Wenn monoenergetische Gammastrahlen zu einem schmalen Strahl kollimiert werden und wenn der Detektor hinter dem Material nur die Gammastrahlen erkennt, die durch dieses Material hindurchgegangen sind, ohne irgendeine Wechselwirkung mit diesem Material, dann sollte die Abhängigkeit eine einfache exponentielle Abschwächung von Gammastrahlen sein . Jede dieser Wechselwirkungen entfernt das Photon aus dem Strahl entweder durch Absorption oder durch Streuung weg von der Detektorrichtung. Daher können die Wechselwirkungen durch eine feste Eintrittswahrscheinlichkeit pro Weglängeneinheit im Absorber charakterisiert werden. Die Summe dieser Wahrscheinlichkeiten wird als Absorptionskoeffizient bezeichnet :
μ = τ (optisch) + σ (Compton) + κ (Paar)
Absorptionskoeffizient
Die Abschwächung der Gammastrahlung kann dann durch die folgende Gleichung beschrieben werden.
I = I 0 .e -μx
, wobei I die Intensität nach der Dämpfung ist, I o die Einfallsintensität ist, μ der lineare Absorptionskoeffizient (cm –1 ) und die physikalische Dicke des Absorbers (cm) ist.
Die in der nebenstehenden Tabelle aufgeführten Materialien sind Luft, Wasser und verschiedene Elemente von Kohlenstoff ( Z = 6) bis Blei ( Z = 82) und ihre Absorptionskoeffizienten sind für drei Gammastrahlenenergien angegeben. Es gibt zwei Hauptmerkmale des Absorptionskoeffizienten:
- Der Absorptionskoeffizient steigt mit zunehmender Ordnungszahl des Absorbers.
- Der Absorptionskoeffizient für alle Materialien nimmt mit der Energie der Gammastrahlen ab.
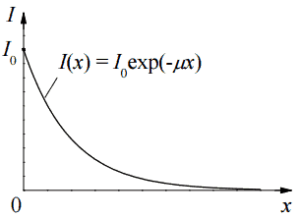 Abhängigkeit der Gammastrahlungsintensität von der Absorberdicke.
Abhängigkeit der Gammastrahlungsintensität von der Absorberdicke.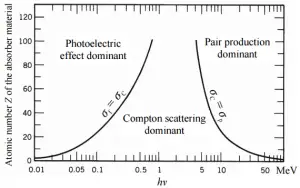 Die relative Bedeutung verschiedener Prozesse der Wechselwirkung von Gammastrahlung mit Materie.
Die relative Bedeutung verschiedener Prozesse der Wechselwirkung von Gammastrahlung mit Materie.
Tabelle der Absorptionskoeffizienten (in cm-1) für verschiedene Materialien bei Gammastrahlenenergien von 100, 200 und 500 keV.
| Absorber | 100 keV | 200 keV | 500 keV |
| Luft | 0,000195 / cm | 0,000159 / cm | 0,000112 / cm |
| Wasser | 0,167 / cm² | 0,136 / cm² | 0,097 / cm² |
| Kohlenstoff | 0,335 / cm² | 0,274 / cm² | 0,196 / cm² |
| Aluminium | 0,435 / cm² | 0,324 / cm² | 0,227 / cm² |
| Eisen | 2,72 / cm² | 1,09 / cm² | 0,655 / cm² |
| Kupfer | 3,8 / cm² | 1.309 / cm² | 0,73 / cm² |
| Führen | 59,7 / cm² | 10,15 / cm² | 1,64 / cm² |