X-rays, also known as X-radiation, refers to electromagnetic radiation (no rest mass, no charge) of high energies. X-rays are high-energy photons with short wavelengths and thus very high frequency. The radiation frequency is key parameter of all photons, because it determines the energy of a photon. Photons are categorized according to the energies from low-energy radio waves and infrared radiation, through visible light, to high-energy X-rays and gamma rays.
Most X-rays have a wavelength ranging from 0.01 to 10 nanometers (3×1016 Hz to 3×1019 Hz), corresponding to energies in the range 100 eV to 100 keV. X-ray wavelengths are shorter than those of UV rays and typically longer than those of gamma rays. The distinction between X-rays and gamma rays is not so simple and has changed in recent decades. According to the currently valid definition, X-rays are emitted by electrons outside the nucleus, while gamma rays are emitted by the nucleus.
Shielding of X-rays
See also: Shielding of Ionizing Radiation
X-ray Attenuation
Source: Wikimedia Commons
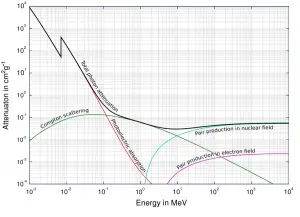
As the high-energy photons pass through material, their energy is decreasing. This is known as attenuation. The attenuation theory is valid for X-rays and gamma rays as well. It turns out that higher energy photons (hard X-rays) travel through tissue more easily than low-energy photons (i.e. the higher energy photons are less likely to interact with matter). Much of this effect is related to the photoelectric effect. The probability of photoelectric absorption is approximately proportional to (Z/E)3, where Z is the atomic number of the tissue atom and E is the photon energy. As E gets larger, the likelihood of interaction drops rapidly. For higher energies, Compton scattering becomes dominant. Compton scattering is about constant for different energies although it slowly decreases at higher energies.
As can be seen, effective shielding of X-rays is in most cases based on use of materials with two following material properties:
- high-density of material.
- high atomic number of material (high Z materials)
However, low-density materials and low Z materials can be compensated with increased thickness, which is as significant as density and atomic number in shielding applications.
A lead is widely used as a X-rays shield. Major advantage of lead shield is in its compactness due to its higher density. A lead is widely used as a gamma shield. On the other hand depleted uranium is much more effective due to its higher Z. Depleted uranium is used for shielding in portable gamma ray sources.
In nuclear power plants shielding of a reactor core can be provided by materials of reactor pressure vessel, reactor internals (neutron reflector). Also heavy concrete is usually used to shield both neutrons and gamma radiation.
In general, the X-rays shielding is more complex and difficult than the alpha or beta radiation shielding. In order to understand comprehensively the way how a X-ray loses its initial energy, how can be attenuated and how can be shielded we must have detailed knowledge of the its interaction mechanisms.
See also more theory: Interaction of X-rays with Matter
See also calculator: Gamma activity to dose rate (with/without shield)
See also XCOM – photon cross-section DB: XCOM: Photon Cross Sections Database
Half Value Layer – X-rays
The half value layer expresses the thickness of absorbing material needed for reduction of the incident radiation intensity by a factor of two. There are two main features of the half value layer:
- The half value layer decreases as the atomic number of the absorber increases. For example 35 m of air is needed to reduce the intensity of a 100 keV X-ray beam by a factor of two whereas just 0.12 mm of lead can do the same thing.
- The half value layer for all materials increases with the energy of the X-rays. For example from 0.26 cm for iron at 100 keV to about 0.64 cm at 200 keV.
Example:
How much water schielding do you require, if you want to reduce the intensity of a 100 keV monoenergetic X-ray beam (narrow beam) to 1% of its incident intensity? The half value layer for 100 keV X-rays in water is 4.15 cm and the linear attenuation coefficient for 100 keV X-rays in water is 0.167 cm-1. The problem is quite simple and can be described by following equation:
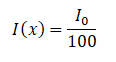 If the half value layer for water is 4.15 cm, the linear attenuation coefficient is:
If the half value layer for water is 4.15 cm, the linear attenuation coefficient is: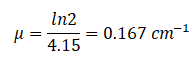 Now we can use the exponential attenuation equation:
Now we can use the exponential attenuation equation: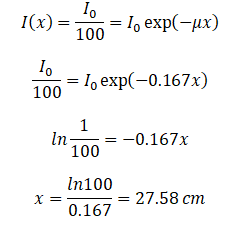
So the required thickness of water is about 27.58 cm. This is relatively large thickness and it is caused by small atomic numbers of hydrogen and oxygen. If we calculate the same problem for lead (Pb), we obtain the thickness x=0.077 cm.
Table of Half Value Layers
Table of Half Value Layers (in cm) for a different materials at photon energies of 100, 200 and 500 keV.
| Absorber | 100 keV | 200 keV | 500 keV |
| Air | 3555 cm | 4359 cm | 6189 cm |
| Water | 4.15 cm | 5.1 cm | 7.15 cm |
| Carbon | 2.07 cm | 2.53 cm | 3.54 cm |
| Aluminium | 1.59 cm | 2.14 cm | 3.05 cm |
| Iron | 0.26 cm | 0.64 cm | 1.06 cm |
| Copper | 0.18 cm | 0.53 cm | 0.95 cm |
| Lead | 0.012 cm | 0.068 cm | 0.42 cm |
We hope, this article, Shielding of X-rays, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about radiation and dosimeters.